
Having never been truly lost while hiking, I imagine that it’s terrifying. In the world of GPS I have an artificial reliance on technology to keep me on the straight and narrow. The entry below is the second part of 3 having to do with navigating mountains and wilderness from “Mountain Scouting, A Hand-Book for Officers and Soldiers on the Frontiers” by Edward S. Farrow, U.S. Army, 1881. Near the end of the explanation Farrow explains through numbers how to calculate your escape. Math is not my strong point so lets just say it took several readings for me to grasp what he was saying. In the coming days I’ll be researching the next and final entry in this series about mountain travel covering ways to signal for help when you are lost in the wilderness.
Mountain Travel
A person may be readily lost in the mountains, and it is never safe to stray off from the camp or command when alone. Should necessity demand it, however, it will prove an excellent rule to take along the rifle and a good supply of ammunition and matches.
Observation goes to show that the horse and other animals, when running on an open ground, will gradually turn their courses to the left. The same is true of man, when lost on the prairies and cut off from all guiding marks or objects—and it is still more the case, when he is lost in the mountains, the irregularities of the route of travel seeming to confuse his ideas of direction and locality. This turning to the left is so rapid with the average lost man, that he will frequently travel in a circle and will, in the course of the day, arrive at the point left in the morning. It should be remembered that, when lost, it is best not to increase the perplexity of the matter by wandering still farther, but set to work to find the way back to known localities. Leave a broad trail for the relieving party to follow and make a great smoke, if possible.
The sense of desolation attending most lost persons is sufficiently overpowering to cause them to lose their presence of mind, to wander widely about and rapidly exhaust their vital powers.
Colonel Dodge relates the following remarkable account:
“When serving in Texas, a soldier of my company became lost while returning to the post from a small village two miles off. A party was sent out to search for him, and on the second or third day came upon him almost naked in a little thicket. As soon as he discovered the party, he bounded off like a deer and was pursued. After an exciting chase he climbed a tree, from which he was taken by force, and with the greatest difficulty—struggling, striking and biting like a wild animal. He was brought back to the post perfectly wild and crazy; confined, watched and attended with the greatest care for over a month before he recovered his mind. He was an excellent man, more than usually intelligent, but I doubt if he ever fully recovered from the shock. He recollected nothing but going a little distance off the road and getting turned around and realizing that he was lost.”
When lost beyond all hope, when there are reasons to believe that a search will not be made, and when it is possible to keep alive for a few days, I believe it a good plan to make for the nearest large water course and follow it down by shore or raft, until the settlements are finally reached. But, before proceeding to this final course be sure that you have well endeavored to answer the following questions,viz.:
A: What is the least distance within which the lost trail lies?
By remaining cool, this may be quite accurately determined by the careful traveler. He knows how long he has been traveling on foot or on horseback, and the average rate of his travel, and he can usually make a fine allowance for loitering, stoppages and remembered zigzags. The lost man may always console himself with the fact that he is nearer the lost trail than he imagines.
B: What is the general direction of the trail?
The traveler should at all times keep in mind the general bearing of prominent landmarks, and the general courses of important streams and ridges. During sunshine, the shadows of trees, etc., will give east and west points. In tropical countries, Orion and Antares give excellent east and west points, and are easily sighted. An almanac, showing the times and bearings of sun rise, sun set, moon rise and moon set for various latitudes, is invaluable to the mountain traveler.
C: On leaving the trail, was the travel to the right or to the left of it?
This question must be answered from memory or the note book, and it is very important that the traveler be able to answer it with proper assurance.
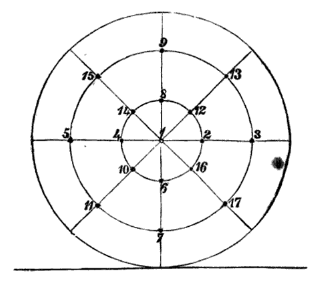
Suppose the traveler is at 1 when he decides that he is lost, and suppose that the trail certainly lies within the distance 1—7; then the circle 7—3—9—5 cuts the trail somewhere. The traveler starting from 1, may first go to 7, and then, in the extreme case, make the entire circuit 7—3—9— 5—7, or travel a distance 7.28 times 1—7 before exhausting his search. Now, suppose that both questions A and B can be answered and that the trail lies within the directions 1—15 and 1—13; then, the trail somewhere cuts the arc 15— 9—13, or possibly the arc 11—7—17.
Produce 7—1—9 until it cuts the tangents to the circle 7—3—9—5—, which are perpendicular to 1—15 and 1—13. Call this distance x. Now this line must cut every trail within and parallel to 1—15 or 1—13 that cuts the circle, and it is furthermore obvious that it must cut every trail that is within and parallel to an intermediate direction.
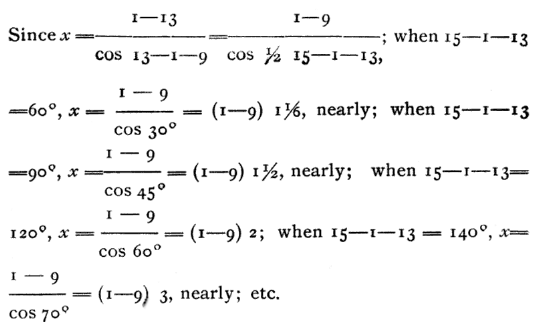
Now, if the traveler is unable to answer question C, he must be prepared to travel from 1 towards 9, a distance equal to x, then back through 1 towards 7, a distance of 2x, or a distance of 3x altogether. If, however, he can answer C, he has no return journey to fear and at worst has to travel towards 9 or 7, a distance equal to x. From this, may be seen the great advantage in being able to answer questions A, B and C conjointly, and how very important it is to know the answer to question B, since x changes so rapidly with the angle 15-1-13.